
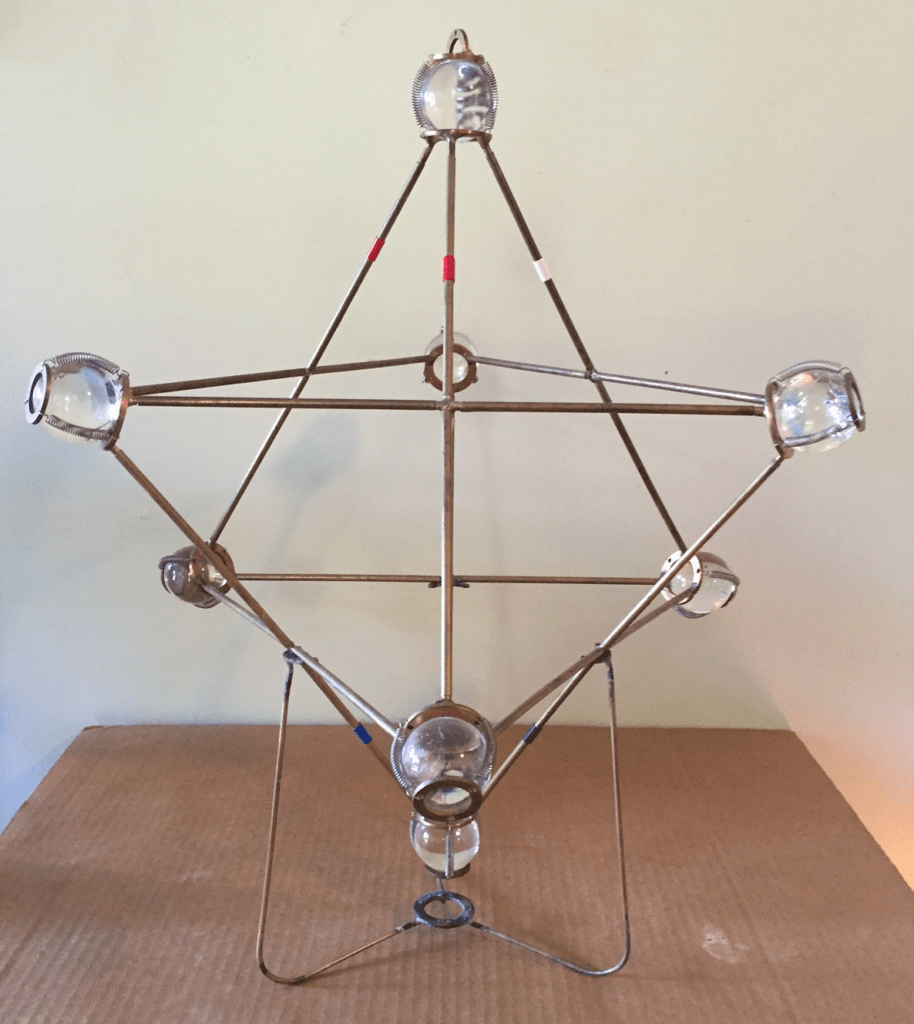
TETRAHEDRON
The Triangle is the most stable structural form, both in two and three dimensions. Triangles exist in a plane, and in three dimensions they are called Tetrahedra. The Tetrahedron, molded from equilateral triangles, is the quintessential form of 3-dimensional space – the most efficient and stable possible expression of an object in space.
Our bodies are both powerfully stable and flexible. Resolving how these two opposing qualities can be simultaneously true is aided by studying both Tensegrity principles and how triangles and tetrahedra formed from tensional and compressional elements interact. When the human body is observed from these perspectives it becomes apparent that they apply almost universally. The body is a free-standing, self-contained, self-propelled, three-dimensional structure that evolved within a competitive ecosystem. It seems likely that, if possible, our lineage would utilize the most efficient structural mechanics available in a three-dimensional setting – the tetrahedron. The section on Tensegrity and Fascia explores this in more detail.
Structural geometries that are more complex than tetrahedra (Polyhedra) are prevalent in our bodies. However, for purposes of modeling tensional and compressional relationships in standing and moving these structures can be reduced to tetrahedra for three-dimensional, or triangles for planes. These reductions make it possible to apply tensegrity biomechanics “on the fly” as we manipulate our bodies to stand and move. Perceiving the expression of load using these geometries can change our tactics and strategies for how we stand and move.
This demo of the “Jitterbug” illustrates some qualities which triangles and tetrahedra express in biological systems
Once one “gets a handle” on how tensegrity biomechanics influence the foundational structural and functional relationships in our bodies, standing and moving take on a fluid weightless quality where gravity is appreciated for its role as an opposing compressional force for our innate tensioning mechanisms. Without gravity, tensegrity would play a much smaller role in mammalian movement dynamics, particularly for bipedal primates like us. This “handle” is the cultivation of the perception of load dynamics in our structure as three-dimensional patterns of tension/compression, interacting at many scales.
The essential concept of Biotensegrity is that load is managed in our structure by trusses – a triangular distribution network that is self-similar at a scale of over more than 6 orders of magnitude. Although load vectors describing the Locomotive Core are presented as lines, loading is in actuality distributed across the entire width and breadth of the body. This is only possible to understand once loading in structure is modeled using the same tools that are employed in Finite Element Analysis, specifically Finite Element Meshes, with which we have only had the computational power to effectively model extremely complex structures in the last few decades. Applying this modeling tool to our human structure in its expresses the vast range of human functional potentials is in its infancy.
TRUSS NETWORKS IN CONNECTIVE TISSUE
Examples from fascial research
Tie back to Tensegrity
TRUSS NETWORKS IN CONNECTIVE TISSUE
Perhaps not coincidentally, the Tetrahedron is also the fundamental form of Sacred Geometry. Here is a post exploring the energetic relationship to Tetrahedra:
ACUPUNCTURE AND 4D GEOMETRY
ANATOMY TRAINS – TOM MEYERS
An excellent, well-established model for understanding human physiology is Anatomy Trains – Tom Meyers. Mastering this model has great utility for users and Manual Therapist of the human body. However, the Meyers model does not emphasize Tensegrity, illustrating structural and functional relationships as tensional “chains” as opposed to interwoven tetrahedra comprising tensional and compressional elements. It is, however, a comprehensive framework that captures many nuances of human structure and function. Although the Anatomy Trains model is presented primarily as a structural model based on tensioning lines in our bodies (a model perhaps closer to a Cable-Stay Bridge as opposed to a tensegrity based Suspension Bridge) it may perhaps have greater utility as a functional model, with the lines representing an aspect of a plane (triangle) perpendicular to, or aligned with a vector of movement or stability, or more simply seen as a reduction of the more complex tensegrity principles underlying our structural mechanics. This writer highly recommends this book to anyone considering developing their Manual Therapy skills or looking to extend their understanding of how tensional networks integrate in our structure and function.
